The goal of this article
The goal of this article is to extend the geography in 3D, including
- Polar coordinates: latitude and longitude
- Cardinal directions: north-south and east-west
into the imaginary planet in 4D.
Contents
- Sine and Cosine
- Euclidean space
- Stereographic projection
- Glome (Hypersphere)
- Hyperspherical coordinates
- Hopf fibration
- Hopf coordinates
Circle
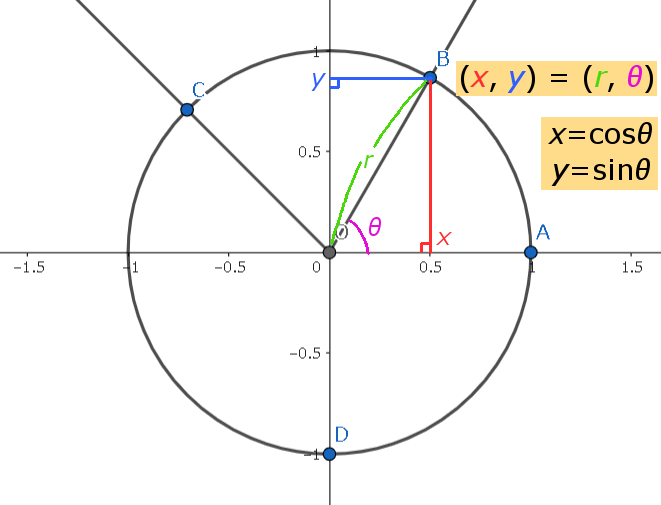
A circle is a closed curve every point of which is equally distant from a fixed point within it.
On the circle, one can move in only one direction; thus, one angular parameter between 0° & 360° can determine any location.
The point of \(\theta\) degrees is on \((\cos \theta, \sin \theta)\).
Sphere
Sphere: a solid figure so shaped that every point on its surface is an equal distance from the center.
The ends of the axis are called Poles.
The mid-section is called the Equator. It is an imaginary circle around the earth that is everywhere equally distant from the two poles.
The Prime Meridian is the line which connects both poles & crosses Greenwich, England. The city is chosen for historical reason.
The surface of Earth is 2-dimensional manifold. That is, any location is expressed with two parameters, and one can move in two perpendicular directions. By defining positive and negative directions, we get four directions: north-south and east-west.
Directions on Earth
The direction to North Pole is called north. Its opposite direction is called south, and it is the direction to South Pole.
The direction of Earth's rotation on any point (except for the poles) is called east, & the opposite direction is called west. The speed of rotation varies from planet to planet.
Coordinates on Earth
Latitude is the angular distance north or south from the Equator measured in degrees.
Longitude is the angular distance east or west from the Prime Meridian.

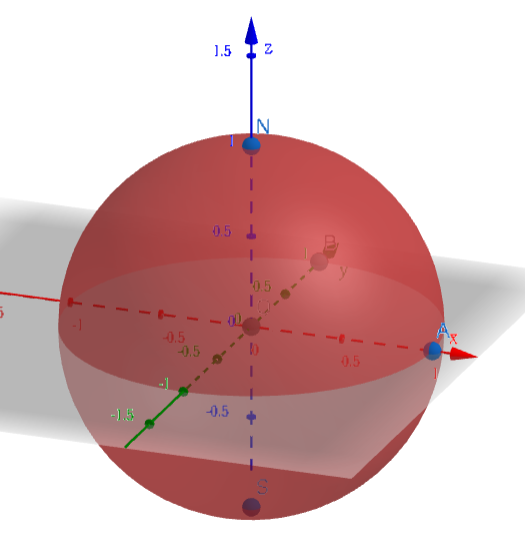
For convenience, we will consider the radius of the earth as 1, the axis of rotation as \(z\)-axis, & the intersection of equator & prime meridian as \((1,0,0)\).
North Pole is \((0,0,1)\); South Pole is \((0,0,-1)\). The equator is on \(xy\)-plane.
The black circle is latitude 45°. Its radius is \(\cos 45°\); it's on the plane \(z= \sin 45°\);
The red circle is the equator; the blue circle is latitude -30°.
Conversion
Generally, the point of \((Lat, Lon)\) is on \((\cos Lat \cos Lon, \cos Lat \sin Lon, \sin Lat)\).
Glome
It's time to move on to 4D space!
In 4D, the equivalent of the sphere is called the hypersphere, or the glome. From now on, all we can do is to imagine. It's hard to imagine it, but it's just all points same distance away from the center, extending to the fourth dimension.
The surface of Earth is 3-dimensional manifold. That is, any location is expressed with three parameters, and one can move in three perpendicular directions.
There are two separate models which explain this.
The "traditional" model
Pole: either end of an axis.
Equator: the mid-section; an imaginary sphere around the planet that is everywhere equally distant from the two poles.
Hypernorth: The direction to the North Pole. The opposite is hypersouth.
Three independent directions: north-south, east-west, hypernorth-hypersouth.
Hyperlatitude: angular distance hypernorth or hypersouth from the equator.
Latitude & longitude: a pair of angular values which show a location on a sphere.
Hyperspherical coordinate system:
Hypernorth Pole is on \((0,0,0,+1)\). Hyperlatitude is +90°.
Hypersouth Pole is on \((0,0,0,-1)\). Hyperlatitude is -90°.
Equator is on xyz-hyperplane.
Stereographic projection:
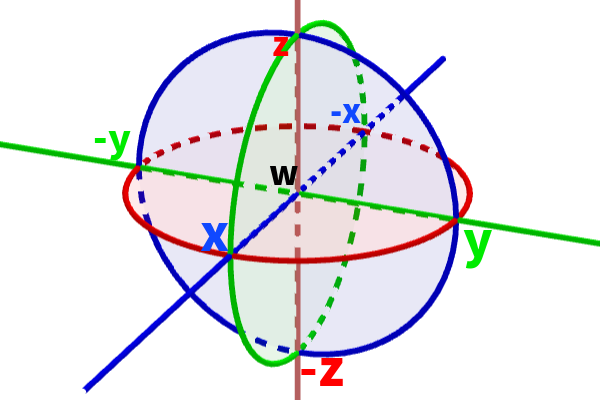
Just like the surface of the sphere can be projected into a 2D map, we can peoject the "surcell" (3D surface) of the glome into a 3D map. In the diagram above, the Hypernorth Pole is in the center, while the Hypersouth Pole is on the point infinitely away from the center.
Note that the stereographic projection shows only the "surcell" (3D surface) of the hypersphere (glome), & the labeled coordinate points are the intersections of the coordinate axes with the surcell of the hypersphere; the coordinate origin is at the center of the hypersphere, & is not represented in the projection.
The "toroidal" model
I apply this in my model of the 4D planet, as it brings more uniqueness & diversity of the 4D world.
The planet has two equators. One is on xy-plane; the other is on zu-plane. I call them South Track & North Track.
The planet has no poles. However, South Track & North Track work just like poles in 3D.
Yin-yang: New terms for directions in 4D space. Can be changed if you're not happy with them.
Three independent directions: north-south, east-west, yin-yang.
From a point on a 4D planet, north is the direction to the closest point on the North Track (R in the figure below). South is the direction to the closest point on the South Track (Q in the figure below) & opposite of north.
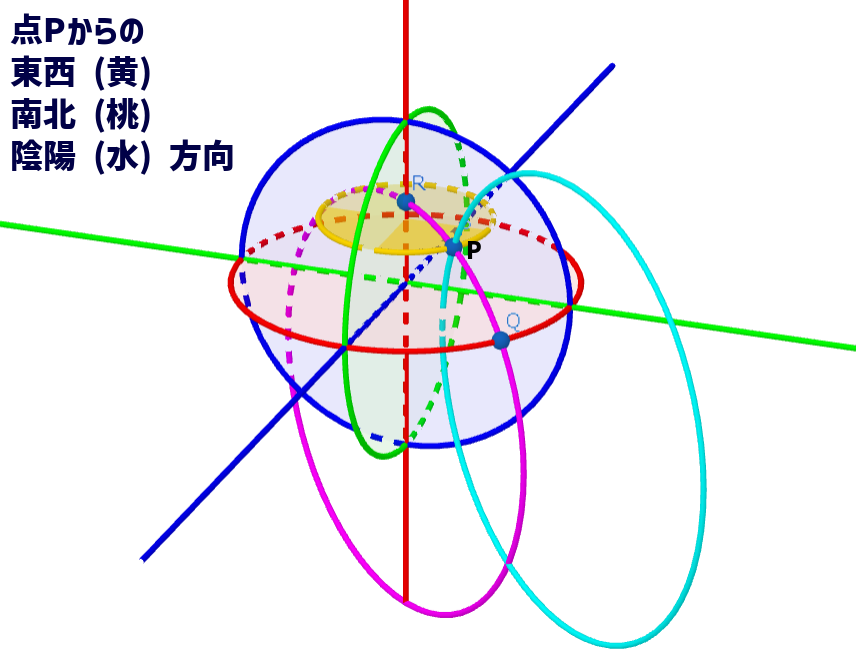
(Yellow: east-west; pink: north-south; cyan: yin-yang)
Latitude: angular distance north or south from the earth's equators. It has a range of only 90 degrees.
Longitude: a pair of angular values, east-west & yin-yang from the prime meridian. Each has a range of 360 degrees. Just like in 3D, it is necessary to artificially determine which point is the 0° of east-west & yin-yang.
Hopf coordinate system:
\(\eta\) is latitude; \(\xi_{1}\) is longitude; \(\xi_{2}\) is colongitude. \(\eta\) moves in a range of only 90°, whereas \(\xi_{1}\) & \(\xi_{2}\) move in a range of 360°.
South Track is on xy-plane. Latitude is 0°.
North Track is on zu-plane. Latitude is 90°.
Every point on the South Track is in the same distance from every point on the North Track, just like poles in 3D. The distance is a quarter lap.
Earth rotates along the xy-plane & zu-plane at the same time, called a double rotation. In my model of the 4D planet, the rotation on the xy-plane is faster than that on the zw-plane.
Note: If the speeds of the two simple rotations are identical, called isoclinic rotation, there are infinitely many ways to define equators.
A group of points where latitude is 45° lies halfway between the two Equators. It partitions a planet into two equal halves. It's in the shape of Clifford torus, which is made of two equal circles.

By east-west and yin-yang rotations, any point moves in the torus surface. A "flat torus" has the property of being able to expand into a rectangle without stretching, so we can draw it on paper as a two-dimensional map connected up-down & left-right, and see how the points rotate and move. Let's take a look!
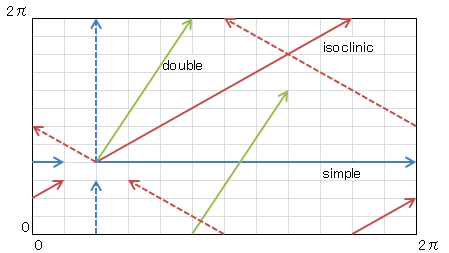
- Double rotation (green) proceeds diagonally along the torus plane. If the angle is \((\alpha, \beta)\), while a point moves for \(\alpha\) in the east, it moves for \(\beta\) in the yang. If the ratio of angles is an irrational number, you will never return to the starting point no matter how many laps you do.
- Simple rotation (blue) has zero angle on one side, so it moves only in the east or yang direction. You will return to the original position after one lap.
- Isoclinic rotation (red) proceeds diagonally, but since both longitudes change by the same magnitude, it returns to the beginning in one lap.
The following image is the stereographic projection of the glome, visualizing some of the torus surfaces. Thin red lines show the paths of points which move as isoclinic rotations.

Move on to the 4D planet article.